Cube Root Of Negative Number
Cube Numbers And Cube Roots
Hither nosotros will acquire about cube numbers and cube roots including what a cube number is, what a cube root is, as well as how to cube a number and how to find the cube root of an integer. You'll too learn how to solve bug by applying cognition of cube numbers.
There are also cube numbers and cube roots worksheets based on Edexcel, AQA and OCR examination questions, forth with further guidance on where to become adjacent if you're yet stuck.
What is a cube number?
A cube number is found when we multiply an integer (whole number) by itself and and so itself once again. These are sometimes called 'perfect cubes'.
We tin cube numbers with decimals places but nosotros practise not refer to these as cube numbers.
A number/variable that is 'cubed' is multiplied by itself three times.
E.g.
A cube number is found when we multiply an integer (whole number) by itself,
Hither are the first
\brainstorm{align*} 1 \times 1 \times 1 &=ane \quad \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } i \text { is a cube number } \\\\ 2 \times two \times 2&=viii \quad \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 8 \text { is a cube number } \\\\ 3 \times three \times 3&=27 \; \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 27 \text { is a cube number } \\\\ 4 \times four \times 4&=64 \; \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 64 \text { is a cube number } \\\\ v \times 5 \times five&=125 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 125 \text { is a cube number } \\\\ half dozen \times half-dozen \times 6&=216 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 216 \text { is a cube number } \\\\ vii \times 7 \times 7&=343 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 343 \text { is a cube number } \\\\ 8 \times 8 \times 8&=512 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 512 \text { is a cube number } \\\\ 9 \times 9 \times 9&=729 \; \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } 729 \text { is a cube number } \\\\ ten \times x \times 10&=yard \quad \quad \quad \quad \quad \quad \quad \quad \text { Therefore } m \text { is a cube number } \end{align*}
A cube number can be represented every bit an array which forms the shape of a cube that has a length 3 units, width 3 units and depth iii units.
East.g.
If we look at
Any cube number will form the shape of a cube.
What is a cube number?
Cubing negative numbers
We can also cube negative numbers.
E.one thousand.
\brainstorm{assortment}{ll} (-v) \times(-five) \times(-5)=-125 & \quad \therefore(-5)^{three}=-125 \\\\ (-7) \times(-7) \times(-7)=-343 & \quad \therefore(-7)^{3}=-343 \end{array}
Y'all will notice that when we cube a negative number we get a negative number.
This is considering a negative number multiplied by a negative number multiplied by a negative number gives us a negative upshot.
Acquire more by reviewing our lesson on negative numbers.
When we cube negative
This is true for all numbers (and variables) and means:
\[(5)^{three} \neq(-v)^{3}\]
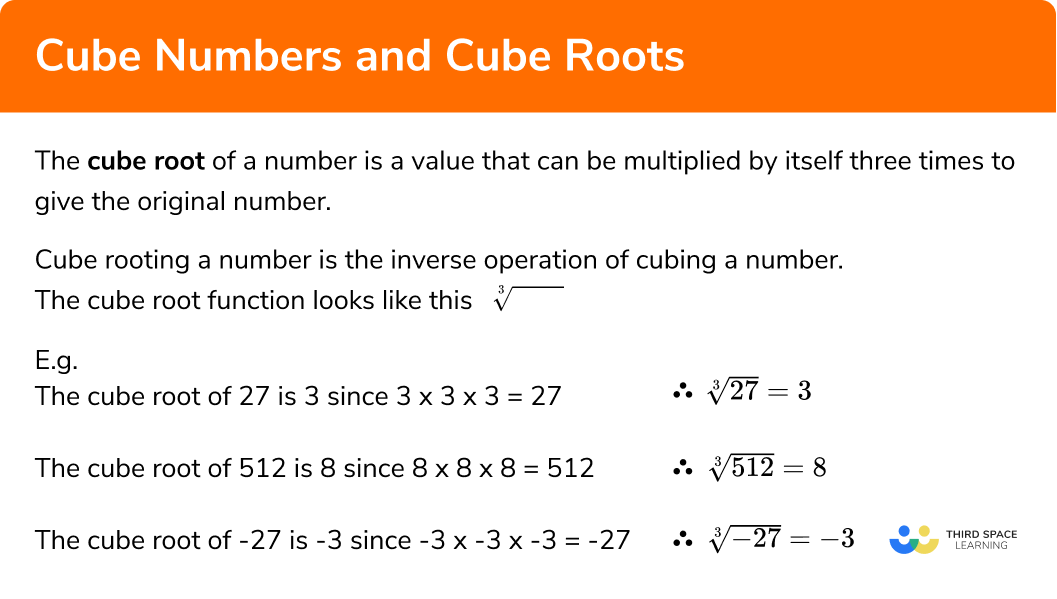
What is a cube root?
The cube root of a number is a value that can be multiplied by itself three times to requite the original number.
A cube root is the inverse functioning of cubing a number.
The cube root function looks similar this
When we cube a positive number we get a positive effect and when nosotros cube a negative number we become a negative event.
And so the cube root of a positive number is besides a positive number, and the cube root of a negative number is besides a negative number.
Due east.g.
\begin{array}{50} \text { As } 3^{iii}=27 \text { the cube root of } 27 \text { is } three & \quad \therefore \sqrt[iii]{27}=3 \\\\ \text { As } 8^{iii}=512 \text { the cube root of } 512 \text { is } eight & \quad \therefore \sqrt[3]{512}=8 \\\\ \text { Every bit} (-3)^{3}= -27 \text { the cube root of} -27 \text { is} -3 & \quad \therefore \sqrt[iii]{-27}=-3 \cease{array}
What is a cube root?
Cube numbers worksheet

Go your complimentary cube numbers worksheet of 20+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREE

Cube numbers worksheet

Get your free cube numbers worksheet of twenty+ questions and answers. Includes reasoning and applied questions.
DOWNLOAD FREE
Key words
Term
A single number (constant) or variable
Due east.g.
in the expression
Coefficient
The number which the variable is being multiplied by
E.g.
in
Integer
A whole number
E.thou.
Index (also called exponent or powers)
The index number is the corporeality of times you multiply a number/variable by itself.
Due east.g.
the index number in
Annotation: the plural of alphabetize is indices
Note: yous will encounter index number as a superscript
Base number
The number/unknown that is beingness multiplied by itself an amount of times
E.g.
the base number in
Advanced vocabulary – only for Additional Maths, A-Level
Real numbers
Whatsoever positive or negative number is chosen a real number. Numbers that are not 'existent' are called imaginary numbers. Integers, decimals, fractions are all examples of existent numbers
E.g.
Imaginary numbers
Numbers that are not existent are chosen imaginary numbers, for example you will notice we cannot find the square root of a negative number (endeavor it on a calculator), this considering it is an imaginary number.. Numbers that contain an imaginary office and real part are chosen circuitous numbers.
Cube numbers and cube roots examples
Example 1
What is
So
Example 2
What is
So
Example 3
What is the cube root of
The cube root of
So
\[\sqrt[three]{64} =four\]
How to use cube numbers and cube roots
- Place whether you need to cube or cube root the number/variable
- Perform the operation
- Clearly state the reply within the context of the question e.k. including units
Now yous volition focus on solving problems using your knowledge of cube numbers and cube roots.
Explain how to solve problems involving cube numbers and cube roots in 3 steps
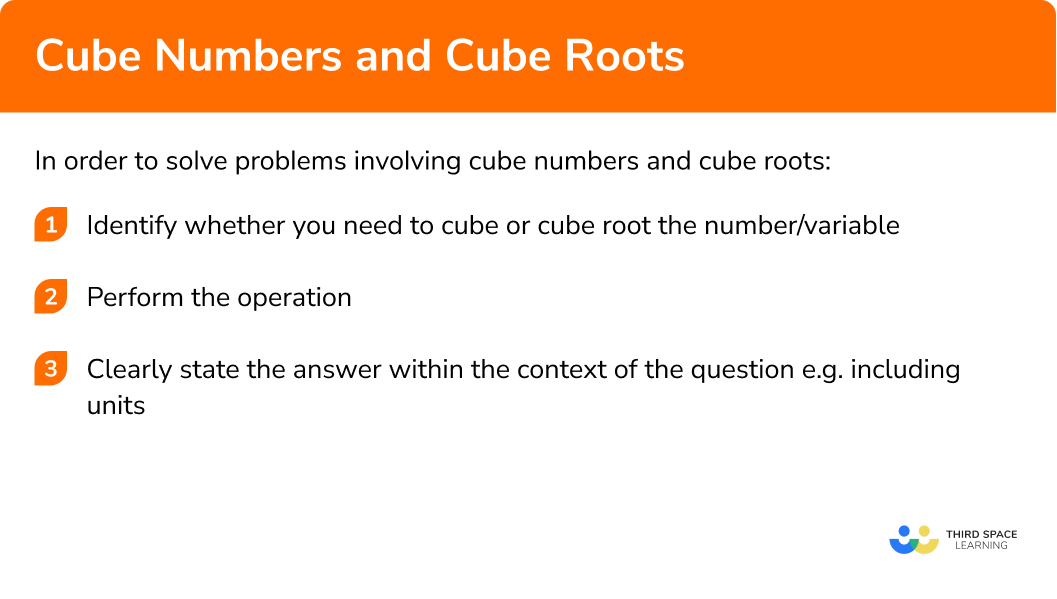
Cube numbers and cube roots problem examples
Example four: noesis of cube numbers
Danny says
- Identify whether yous demand to cube or cube root the number/variable
The question focus is on cubing
two Perform the operation
\brainstorm{array}{fifty} ii \times two \times ii =2^{3} =eight \finish{array}
3 Clearly state the respond within the context of the question
Danny is wrong because
The mistake Danny made was he did
Example 5: problem solving with cube roots
Ava says the cube root of an integer is always smaller than the original number. Testify Ava is wrong.
Place whether you need to cube or cube root the number/variable
You are looking for a relationship between the cube root of an integer and the original number. It will aid to write down your cube numbers and their cube roots.
\brainstorm{array}{l} \sqrt[iii]{1000}=10 \\\\ \sqrt[3]{729}=ix \\\\ \sqrt[3]{512}=eight \\\\ \sqrt[3]{343}=7 \\\\ \sqrt[three]{216}=half dozen \\\\ \sqrt[3]{125}=5 \\\\ \sqrt[three]{64}=4 \\\\ \sqrt[3]{27}=3 \\\\ \sqrt[iii]{eight}=2 \\\\ \sqrt[3]{1}=ane \end{array}
Clearly state the answer within the context of the question
\[\sqrt{1}=1\]
The respond here is not smaller than the original number.
Example half-dozen: solving problems involving cube numbers
The sum of 2 cube numbers is
Identify whether yous need to cube or cube root the number/variable
Remember sum means add. Therefore yous are looking for two cube numbers that add together to make
It will assist here to list the cube numbers up to
The Cube Numbers:
You lot at present need to pick two of these numbers that when added together make
Clearly state the answer inside the context of the question
The ii cube numbers are
Instance 7: cube numbers within a 3D polygon
A cube has a volume of
Identify whether you need to cube or cube root the number/variable
The volume of a cube is found past multiplying the length, width and peak together. For a cube the length, width and tiptop are the aforementioned length.
Therefore yous are looking for a number that when multiplied past itself three times (or cubed) is equal to
Therefore yous need to find the cube root of
Clearly state the answer within the context of the question
Therefore the length of the cube is
Instance eight: cube numbers within a 3D polygon
Length of ane side of a cube is
Identify whether you need to cube or cube root the number/variable
The book of a cube is institute past multiplying the length, width and top together. For a cube the length, width and acme are the aforementioned length.
Therefore you are are going to multiply the side length by itself three or 'cube' the length.
Therefore you need to cube
Clearly state the answer within the context of the question
Therefore the volume of the cube is
Example nine
Lexi says "when yous add three consecutive cube numbers, the answer is always odd."
Is Lexi correct? Explain your respond.
Place whether you need to cube or cube root the number/variable
To prove Lexi wrong we simply need to discover one example where she is incorrect, this is sometimes known as proof past contradiction.
Therefore we are looking for
\[1, \quad 8, \quad 27, \quad 64, \quad 125, \quad 216, \quad 343, \quad 512, \quad 729, \quad yard \]
You are now looking for one example where three of these numbers when added together make an even number.
For case:
Clearly state the reply inside the context of the question
Lexi is wrong because
Common misconceptions
- Cube numbers
Incorrect understanding of cubing a number
Eastward.thou.
non
- Cube roots
Not recognising that a negative number cube rooted is negative
E.g.
\sqrt[3]-viii = -2
Practice cube numbers and cube roots questions




10^{3}=10 \times 10 \times x = 1000




six \times 6 \times 6 = 216 \quad \text{ therefore } \quad \sqrt[three]{216} = vi




(-7)^{3} = (-7) \times (-7) \times (-7) = -343




(-1) \times (-1) \times (-1) = -1 \quad \text{ therefore } \quad \sqrt[3]{-one}=-one




The cube numbers up to 65 are ane, 8, 27, 64.
The 2 that add up to 65 are 1 and 64.




Nosotros find the book of a cube by multiplying the length, the width and the superlative together.
These are all equal for a cube and so we need to find a number that, when multiplied by itself 3 times, gives us 512.
Therefore we need to find the cube root of 512.
\sqrt[3]{512}=8
Cube numbers and cube roots GCSE questions
1. Work out the value of:
(a) 3^{3}
(b) five \times two^{three}
(c) 6^{3} − iii^{3}
(3 Marks)
Testify reply
(a) 3 \times iii \times 3
27
(one)
(b) five \times viii
40
(ane)
(c) 216 – 27
189
(i)
2. Here is a list of numbers:
1000 \quad \quad 18 \quad \quad 8 \quad \quad 64 \quad \quad seven \quad \quad 144 \quad \quad one \quad \quad xix
(a)List the cube numbers
(b)Which number is a square and cube number?
(2 Marks)
Bear witness answer
(a) k, 8 , 64 and 1
(one)
(b) 64
(1)
three. Find the value of:
(a) \sqrt[3]{729}
(b) \sqrt[3]{-1}
(c) \sqrt[iii]{-8}
(3 Marks)
Bear witness answer
(a) nine
(1)
(b) -1
(1)
(c) -two
(1)
iv. Simplify the following expression
5^{3} \times \sqrt[three]{x^{3}}
(2 Marks)
Show answer
125 10
Correct coefficient
(1)
Correct x (or x^{1} )
(ane)
Learning checklist
You have now learned how to:
- Calculate cube numbers up to x × x × 10
- Use positive integer powers and their associated real roots
- Recognise and use the cube numbers
- Apply properties of cubes to a context
Still stuck?
Prepare your KS4 students for maths GCSEs success with Tertiary Space Learning. Weekly online one to 1 GCSE maths revision lessons delivered by skilful maths tutors.

Detect out more well-nigh our GCSE maths revision programme.
Cube Root Of Negative Number,
Source: https://thirdspacelearning.com/gcse-maths/number/cube-numbers/
Posted by: rodgersalsoned.blogspot.com


0 Response to "Cube Root Of Negative Number"
Post a Comment